Why is the price right?

Returning to our old arbitrage argument, here is what the buyer of the derivative can do if the price is set at p^', which is less than the value p as specified by equation .

1.

he would buy 1 unit¹² of derivative at time zero and pay p^' dollars to the seller.

2.

also at time zero, sell the portfolio with content 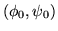$(\phi_{0},\psi_{0})$ and receive an amount p.

3.

put the difference p-p^'>0 in a bank and at time T, he would get 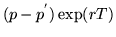$(p-p^{'})\exp(rT)$ dollars.

4.

at time T, he would buyback the original portfolio, using money from the worth of the derivative at time T, no matter whether the stock price is up or down!¹³

5.

the conclusion is: using this method, the buyer loses nothing by buying the derivative; moreover, he also gains a risk free profit of 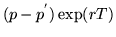$(p-p^{'})\exp(rT)$ dollars at time T!

Obviously, we can also reverse the argument if p^'>p; therefore p^'must be equal to p in order to prevent arbitrage.