• Week 1
• Week 2
• Week 3
• Week 4
• Week 5
• Week 6
• Week 7
• Week 8
• Week 9

Here is the plan as we see it now, it is, of course, subject to revision as the course progresses.

Week 1 - The Discovery of Neutron Stars

Problem 1 - The Eddington Luminosity

There is a natural limit to the luminosity a gravitationally bound object can emit. At this limit the inward gravitational force on a piece of material is balanced by the outgoing radiation pressure. Although this limiting luminosity, the Eddington luminosity, can be evaded in various ways, it can provide a useful (if not truly firm) estimate of the minimum mass of a particular source of radiation.

1. Consider ionized hydrogen gas. Each electron-proton pair has a mass more or less equal to the mass of the proton (m_p) and a cross section to radiation equal to the Thompson cross-section (σ_T).
2. The radiation pressure is given by outgoing radiation flux over the speed of light.
3. Equate the outgoing force due to radiation on the pair with the inward force of gravity on the pair.
4. Solve for the luminosity as a function of mass.

Problem 2 - Minimum Masses

The observations of Sco X-1 can give a lower limit on the mass of the sources if they are gravitationally bound.

The source discovered by Giacconi et al. is now known as Sco X-1.

1. What is the most likely distance to Sco X-1 given its location on the sky?
2. At this distance given the flux estimate in the Giacconi et al., what is the luminosity of Sco X-1?
3. What is the minimum mass of Sco X-1?

Week 2 - Rotation-Powered Neutron Stars

Problem 1 - Spinning Neutron Stars

We will estimate how quickly we would expect neutron stars to spin, how much energy is stored in their spin and other interesting facts about spinning neutron stars.

1. The sun rotates every 24-30 days depending on latitude. How quickly would it rotate if it were compressed to 10km in radius while conserving its angular momentum? Its current radius is 7 x 10¹⁰ cm.
2. How fast could a neutron star rotate without breaking up? Consider the neutron star to be 1.4 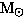and have a radius of 10 km and compare the centripetal acceleration of a bit of material on the surface to the gravitational acceleration.
3. How much angular momentum and rotational energy does a neutron star have? Use

   I ≈ 0.21

   M R2 1-2 G M R c2

and a spin period of break-up, 1.6 ms, 33 ms and 6 s.

Problem 2 - Original Spin

If you know the age of a neutron star, its current period and its period derivative, you can estimate its original spin.

1. Using the results from the lectures, derive a formula for P₀ in terms of the age, current period and period derivative of a pulsar.
2. The table below lists pulsars that are associated with historical supernovae. Complete the table by calculating the original spin of these neutron stars.

   Name	Age [yr]	Period [s]	P-dot [10-15 s s-1]	P0 [s]
   B0531-21	949	0.0331	422.69
   B0540-69	1000	0.050	480
   B1951+32	64000	0.0395	5.8
   J0205+6449	822	0.06568	193

3. Why do we know the age of the first and last pulsars so accurately?

Week 3 - The Structure of Neutron Stars

Problem 1 - Newtonian Polytropes

1. Consider a star of mass, M, and radius, R. Construct by dimensional analysis a characteristic pressure and a characteristic density from these quantities and Newton's constant, G.
2. A polytropic equation of state is a power-law relationship between pressure and density, P = K ρ^α. Substitute the characteristic pressure and density into the polytropic equation of state to derive a mass-radius relation.
3. Which values of α have special properties? What are they?

Problem 2 - Central Pressures

We will calculate the central pressure of a incompressible star in Newtonian physics and general relativity.

1. Use the General Relativistic equations of hydrostatic equilibrium to determine the central pressure of a star of mass M and radius R. The material is incompressible, i.e. its density is constant. After integrating (9) from the center where the enclosed mass, u, vanishes, it is easiest to integrate (10) from the surface where the pressure vanishes. Write your answer as a function of M and R by eliminating the constant density from the result.
2. By dimensional analysis, figure out where the factors of G and c appear in the General Relativistic equations of hydrostatic equilibrium.
3. Derive the Newtonian equations of hydrostatic equilibrium by taking the limit of c → ∞.
4. Redo the pressure calculation in Newtonian physics.
5. By dimensional analysis, figure out where the factors of G and c appear in your answer to (1).
6. Check your answers by taking the limit of c → ∞ for your answer to (5) and comparing it with the answer to (4).
7. What is the minimal radius for a constant-density star of a given mass? What is the maximal mass for a star of a particular density? What is the maximal mass for a star at nuclear density, 10¹⁵ g cm^-3?

Problem 3 - Neutron Star Masses

Calculate from dimensional analysis the typical mass of a neutron star.

1. Use the characteristic density and pressure of a star that you derived in Problem 1. Neutron stars have relativistic neutrons so the pressure is about the density times c². Use this to derive a relationship between the mass and radius of the star.
2. A relativistic degenerate gas has a density of one particle in a cube a Compton wavelength on a side. Combine this with the result from Part 1 to solve for the mass of the star.

Week 4 - Neutron-Star Cooling and Heating

Problem 1 - Thermodynamics and General Relativity

In general relativity if two bodies are in thermodynamic equilibrium,

T1 1 + z1

=

T2 1 + z2.

We can exploit this relationship along with Kirchoff's law to derive some interesting facts about how light travels from a neutron star to our telescopes. You can experiment with neutron-star lensing with this Java Applet.

1. Because everything is in thermodynamic equilibrium, we can safely assume that the neutron star of mass M and radius R emits as a blackbody at a temperature T. Calculate the total power emitted from the neutron star surface in the frame of the neutron star surface.
2. Calculate the total power received at infinity. Let the redshift of the surface be z.
3. Let the space surrounding the star be filled with blackbody radiation in thermal equilibrium with the surface of the neutron star. You can imagine that the neutron star is in a gigantic thermos bottle. Let T_∞ be the temperature of this blackbody radiation measured at infinity (i.e. z=0). What is T_∞?

Now here comes Kirchoff's law: in thermodynamic equilibrium a body emits as much as it receives.

4. How much power does the neutron star absorb from the blackbody at infinity? This is the product of the surface area of the neutron star with the flux per unit area of the blackbody radiation.

A conundrum: compare the answer to (2) with the answer to (4). They differ. Does the neutron star cool down because (2) is greater than (4)?

The neutron star can't cool down because it is already in equilibrium, so one of our assumptions must be wrong.

5. It turns out that the most innocuous sounding assumption is incorrect. The power that the neutron star absorbs is the product of its apparent surface area with the flux per unit area of the blackbody radiation. Let the apparent radius be R_∞ and recalculate the answer to (4).
6. Equate (2) and (5) and solve for R_∞.

R_∞ ≠ R because in the vicinity of a neutron star light does not travel in a straight line. One can also derive the value of R_∞ by solving for a null geodesic that is tangent to the surface of the neutron star.

7. What is the minimum value of R_∞ for a constant value of M? You will need to know that

   1+z =

   1 (1 - 2 G M/R c2)1/2

   What is the value of R? Call this radius R_γ.
8. Prove the size of the image of the neutron star must decrease or remain the same as the radius of the neutron star decreases. Use the fact that rays that we ultimately see remain outgoing throughout their journey to us (otherwise by symmetry they would hit the surface a second time).
9. What happens to the size of the image of the star if the radius of the star is less that R_γ?
10. The calculation of the apparent radius of the star from thermodynamics hinges on the assumption that the outgoing flux from the surface reaches infinity. For R < R_γ, the size of the image no longer increases while thermodynamics says it should, so we must conclude that for radii less than R_γ initially outgoing photons can become incoming photons.

    From these arguments and spherical symmetry speculate what might happen to a photon emitted precisely at R_γ tangentially, i.e. neither ingoing or outgoing.

11. Calculate how much radiation a star whose radius is less than R_γ will absorb.

The answer to (11) falls short of (2) again. We know that the star can't heat up, so an assumption must be wrong. Within R_γ not every photon emitted can escape to infinity, many photons return and hit the surface.

12. Using the answers to (2) and (11), calculate the fraction of the outgoing photon flux emitted from the surface that manages to escape.
13. Use the fact that a blackbody emits isotropically to determine the opening angle of the cone into which the escaping photons are emitted. This region is symmetric around the radial direction.
14. Pat yourself on the back. You have derived many of the quirky things about the Schwarschild metric (the metric that surrounds a spherically symmetric mass distribution). List the key assumptions that you have made to make this derivation work.

Problem 2 - Light-Envelope Neutron-Star Cooling

The presence of hydrogen in the atmosphere of a neutron star strongly affects the emission of the surface of the star and possibly how the star cools.

1. Calculate the maximum possible density of pure degenerate ionized hydrogen gas (non-degenerate protons and degenerate electrons). What happens above this density?
2. Assume that the electrons dominate the pressure, what is the pressure at this critical density?
3. Assume that the gravitational acceleration is constant in this thin layer and is given by g_s,1410¹⁴ cm s^-2. What is the column density of the layer?
4. Assume that the stellar radius is R₆ 10⁶ cm. How much hydrogen can you pile onto a neutron star?
5. Assume that the cross section to x-rays per electron-proton pair is given by the Thomson cross-section. What is the column density of an optically thick layer of hydrogen (τ=1)?
6. The conductivity of the surface layers of a neutron star is inversely proportional to the atomic number of the nuclei. How does the surface luminosity of neutron star change if you pour hydrogen onto its surface?

Week 5 - Accreting Neutron Stars

Problem 1 - Accretion

1. Let's use Newtonian gravity for simplicity here. How much kinetic energy does a gram of material have if it falls freely from infinity to the surface of a star of mass M and radius R?
2. How much energy is released if a gram of material falls from a circular orbit just above the stellar surface onto the stellar surface? To put it another way, what is the kinetic energy of the material in the circular orbit?
3. Hydrogen burning releases about 6 x 10¹⁸ erg/g. How does accretion of hydrogen onto a neutron star (R=10km, M=1.4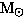) differ from accretion onto a white dwarf (R=10000 km, M=0.6)?
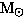4. What is the total about of energy released per gram of material as it falls from infinity to the surface of a neutron star? How many grams of material would have to fall each second on the neutron star to generate an Eddington luminosity through accretion? This is called the Eddington accretion rate.

Problem 2 - Bursts

We will try to model Type-I X-ray bursts using a simple model for the instability. We will calculate how much material will accumulate on a neutron star before it bursts.

1. Let us assume that the star accretes pure helium, that the temperature of the degenerate layer is constant down to the core (T_c), how much luminosity emerges from the surface of the star? (You shouldn't have to derive this formula (I gave it to you in class).
2. Let us assume that the helium layer has a mass, dM, and that the enregy generation rate for helium burning is given by

   ε3α = 3.5 x 1020 T9-3 exp(-4.32/T9) erg s-1 g-1

   where T₉=T/10⁹K. The energy generation rate is a function of density too, but let's forget about that to keep things simple. How much power does the helium layer generate as a function of dM?
3. Equate your answer to (1) to the answer to (2) and solve for dM. This is the thickness of a layer in thermal equilibrium.
4. Let's assume that the potential burst starts by the temperature in the accreted layer jiggling up by a wee bit. If the surface luminosity increases faster with temperature than the helium burning rate, then the layer is stable. Calculate dL_surface/dT and dP_helium/dT.
5. Calculate the value of dM for which dP_helium/dT exceeds dL_surface/dT and the layer bursts.
6. Equate your value of dM in (3) and (5) and solve for T. What is dM? How long will it take for such a layer to accumulate if the star is accreting at one-tenth of the Eddington accretion rate?

Week 6 - Exoplanets I

Problem 1 -

Week 7 - Exoplanets II

Week 8 - Exoplanets III

Week 9 - Exoplanets IV

Week 10 - Cosmology I

Week 11 - Cosmology II

Week 12 - Cosmology III

Problem 1 -

Week 13 - Cosmology IV