Reading for next week: the following from Knight (plus other parts of chapters 10 and 11 if you like):

Chapter 10.1
Chapter 10.2: section called "Kinetic and Potential Energy"
Chapter 10.3: section called "Conservation of Mechanical Energy"
Chapter 10.5: read about the potential energy stored in a spring to do the Mastering Physics question about springs
Chapter 10.7: read up to equation 10.39
Chapter 11.1

Mastering Physics: due Tuesday at 10:00pm

Written Questions:

Problem 1:



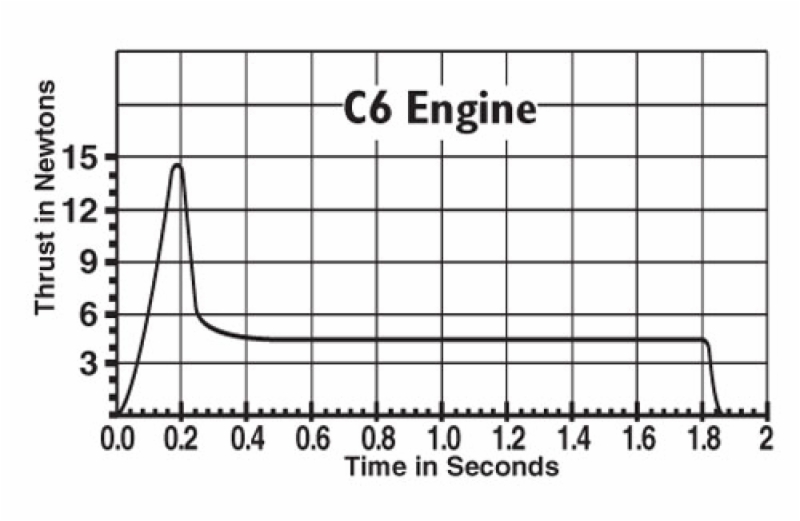
For her 12th birthday, Abby gets a Barbie doll (from a kindly aunt) and a model rocket kit (from her parents, who know she's more into science). Abby decides to see how high Barbie can fly, so she rigs up a rocket pack with the C6 model rocket engine that came in her kit. The graph above shows the thrust force for this engine as a function of time after the rocket is ignited, but you can approximate this by a function that is F(t) = t × 75 N/s  from t=0 to t=0.2s and F(t) = 5 N from 0.2s to 1.8s. If we assume Barbie and her rocket pack have a constant total mass of 0.2kg during the flight, and we ignore air resistance, how high does Barbie get?


Bonus Problem:

Suppose the sea cucumber in from last week's question actually ejects its viscera over a period of time, and that the speed of the ejected material is always 1m/s relative to the sea cucumber. Determine the final speed of the sea cucumber relative to the shuttle.

Extra Practice Question (because you really can't get enough sea cucumber physics questions)
(don't hand this in)

During her Science One trip to Bamfield, Mildred befriends a sea cucumber named Doug and decides to take Doug home as a pet. When they get on the ferry to go back home, Mildred takes Doug out onto the deck of the ferry to show him the view. Unfortunately, just when they are looking out over the railing, the ship's horn blasts, causing Doug to become agitated and expel his viscera.  Surprised by this development, Mildred accidentally lets go of Doug, and he falls towards the water, from an initial height of 10m.
If the expulsion of the viscera results in a horizontal recoil force on Doug that increases as F = (1 N/s²) t² until t=0.5s and then decreases as F = 0.5N/s (1s - t) until t=1s when the viscera have finished being expelled, determine how far Doug (mass 100g, initially stationary relative to the water) travels horizontally before he hits the water (and lives happily ever after after regenerating his internal organs).